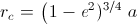Conical Sections: A Deeper Look Part 2
This post continues from the previous post.
The previous post presented some very fundamental features of a cone and the ellipse that is created by a cutting plane going through it at an angle. I would now like to pick a particular cone and cutting angle to use for a numerical example. At this point, any cone-angle combination is equally suitable. However, perhaps by examining some other features of a conic section, a particular section will suggest itself for use as a numerical example.
Some conditions:
First, the cutting angle, α, must satisfy the following condition: 0 < α < arctan(γ).
If the angle is too big or too small, the resulting section will not be an ellipse; it will be a circle, parabola, or hyperbola.
Second, at the bottom of the previous post, I determined that the value of α for which ru is a minimum is α = arctan(1/γ).
So, I will restrict my examination to right cones for which γ > 1.
Third, because I am hoping this exploration will reveal some information that may provide further insight applicable to elliptical motion, I am going to impose one more condition:
a circular section going through the same right cone, of equal area of the ellipse, must be at the same height on the cone as the mid-point of the ellipse.
Area of a circle: π r ²
Area of an ellipse: π ab
Therefore, hqa = γ √(ab) = hc + ro sin(α)
After some algebraic manipulation, this reduces to
cos(α) = (1 - e²)(1/4)
Which, in turn, leads to cos(α) = (1/γ).
So now a condition exists to define a particular class of elliptical section, which I am calling a qa-section (an elliptical section produced such that its equal-area circle is at the mid-point of the ellipse). Following are some properties of these qa-sections (including parameters from my previous post which can be simplified under these conditions).
However, even after applying these restrictions, an infinite number of sections is still possible. Perhaps a graph will illuminate some feature I can use. On the following image are two graphs: αqa and αrumin versus γ.
αqa (the red line) is α for a qa-section (= arccos(1/γ)).
αrumin (the blue line) is the value of α for which ru is a minimum.
These lines intersect at only one point; this point suggests the cone-cutting plane I will use for numerical examples.
In fact, this point of intersection occurs when arccos(1/γ) = arctan(1/γ)
γ is then found as the solution to the following equation: γ 4 - γ ² - 1 = 0
This equation has four solutions:
These solutions correspond to γ = +/- 1.272019649514069 and +/- 0.786151377757i.
The imaginary roots and the negative real root are meaningless in the present context; only the real, positive, solution is applicable:
γ = 1.272019649514069
Alternately, these roots could have been found by forming the Companion Matrix for this polynomial and computing its eigenvalues and eigenvectors. The eigenvalues are the roots of the original polynomial. The Companion Matrix is
Two eigenvalues are +/- 0.7861513777574233i, and the associated eigenvectors are
The other two eigenvalues are +/- 1.272019649514069, and the associated eigenvectors are
So the roots have been confirmed.
This cone (γ = 1.272019649514069), and all the properties that follow from it, will be used for numerical examples going forward.
Before closing this post, I would like to point out that simply projecting a circle onto a plane inclined at an angle of 0.666239432488 radians would not produce an ellipse of eccentricity e = 0.786151377754. In fact, you would produce an ellipse, but not one of this eccentricity.
To confirm this assertion, y-coordinates of a circle have been multiplied by cos(α) and tabulated in a table in a previous post (Blog Post: Ellipse Sample Datapoints), under the column labeled ycon. Note that the values tabulated in this column do not product an ellipse of eccentricity 0.786151377754. In fact, an ellipse of eccentricity 0.618033988747 is produced. To produce an ellipse of the same eccentricity as the one produced by the conic section--and a cutting plane of the same angle, α--the ycon values have to be multiplied by an additional factor of cos(α).
The previous post presented some very fundamental features of a cone and the ellipse that is created by a cutting plane going through it at an angle. I would now like to pick a particular cone and cutting angle to use for a numerical example. At this point, any cone-angle combination is equally suitable. However, perhaps by examining some other features of a conic section, a particular section will suggest itself for use as a numerical example.
Some conditions:
First, the cutting angle, α, must satisfy the following condition: 0 < α < arctan(γ).
If the angle is too big or too small, the resulting section will not be an ellipse; it will be a circle, parabola, or hyperbola.
Second, at the bottom of the previous post, I determined that the value of α for which ru is a minimum is α = arctan(1/γ).
So, I will restrict my examination to right cones for which γ > 1.
Third, because I am hoping this exploration will reveal some information that may provide further insight applicable to elliptical motion, I am going to impose one more condition:
a circular section going through the same right cone, of equal area of the ellipse, must be at the same height on the cone as the mid-point of the ellipse.
Area of a circle: π r ²
Area of an ellipse: π ab
Therefore, hqa = γ √(ab) = hc + ro sin(α)
After some algebraic manipulation, this reduces to
cos(α) = (1 - e²)(1/4)
Which, in turn, leads to cos(α) = (1/γ).
So now a condition exists to define a particular class of elliptical section, which I am calling a qa-section (an elliptical section produced such that its equal-area circle is at the mid-point of the ellipse). Following are some properties of these qa-sections (including parameters from my previous post which can be simplified under these conditions).
Summary of Features for a qa Elliptical Section
However, even after applying these restrictions, an infinite number of sections is still possible. Perhaps a graph will illuminate some feature I can use. On the following image are two graphs: αqa and αrumin versus γ.
αqa (the red line) is α for a qa-section (= arccos(1/γ)).
αrumin (the blue line) is the value of α for which ru is a minimum.
These lines intersect at only one point; this point suggests the cone-cutting plane I will use for numerical examples.
In fact, this point of intersection occurs when arccos(1/γ) = arctan(1/γ)
γ is then found as the solution to the following equation: γ 4 - γ ² - 1 = 0
This equation has four solutions:
These solutions correspond to γ = +/- 1.272019649514069 and +/- 0.786151377757i.
The imaginary roots and the negative real root are meaningless in the present context; only the real, positive, solution is applicable:
γ = 1.272019649514069
Alternately, these roots could have been found by forming the Companion Matrix for this polynomial and computing its eigenvalues and eigenvectors. The eigenvalues are the roots of the original polynomial. The Companion Matrix is
Two eigenvalues are +/- 0.7861513777574233i, and the associated eigenvectors are
The other two eigenvalues are +/- 1.272019649514069, and the associated eigenvectors are
So the roots have been confirmed.
This cone (γ = 1.272019649514069), and all the properties that follow from it, will be used for numerical examples going forward.
Some Numbers for the qa Elliptical Section for γ = 1.272019649514069
(a = 1)
γ = 1.272019649514069
α = 0.666239432488 radians
e = 0.786151377754
ru = 0.381966011254
rl = 1.61803398875
ro = 0.618033988738
rc = 0.485868271774
hc = 0.618033988764
b = 0.618033988764
hqa = 1
rqa = 0.786151377767
cos(α) = 0.78615137776
sin(α) = 0.618033988746 = cos²(α)
tan(α) = 0.78615137776
Note that the expression under the radical returns itself:
0.618033988738 = √(1 - √ (1 - e²))
0.618033988738 = √(1 - √(1 - √ (1 - e²)))
etc.
This could go on forever.
In other words, this number satisfies the infinite expression
x = √(1 - √ (1 - √ (1 - √ (...))))
γ = 1.272019649514069
α = 0.666239432488 radians
e = 0.786151377754
ru = 0.381966011254
rl = 1.61803398875
ro = 0.618033988738
rc = 0.485868271774
hc = 0.618033988764
b = 0.618033988764
hqa = 1
rqa = 0.786151377767
cos(α) = 0.78615137776
sin(α) = 0.618033988746 = cos²(α)
tan(α) = 0.78615137776
Note that the expression under the radical returns itself:
0.618033988738 = √(1 - √ (1 - e²))
0.618033988738 = √(1 - √(1 - √ (1 - e²)))
etc.
This could go on forever.
In other words, this number satisfies the infinite expression
x = √(1 - √ (1 - √ (1 - √ (...))))
Before closing this post, I would like to point out that simply projecting a circle onto a plane inclined at an angle of 0.666239432488 radians would not produce an ellipse of eccentricity e = 0.786151377754. In fact, you would produce an ellipse, but not one of this eccentricity.
To confirm this assertion, y-coordinates of a circle have been multiplied by cos(α) and tabulated in a table in a previous post (Blog Post: Ellipse Sample Datapoints), under the column labeled ycon. Note that the values tabulated in this column do not product an ellipse of eccentricity 0.786151377754. In fact, an ellipse of eccentricity 0.618033988747 is produced. To produce an ellipse of the same eccentricity as the one produced by the conic section--and a cutting plane of the same angle, α--the ycon values have to be multiplied by an additional factor of cos(α).
Labels: conical section, ellipse, elliptical section